Andrew Wiles

Fermat’s Last Theorem

Parama Dutta

Jun 15, 2013 • 4 min read

Google Doodle depicting Fermat’s Last Theorem

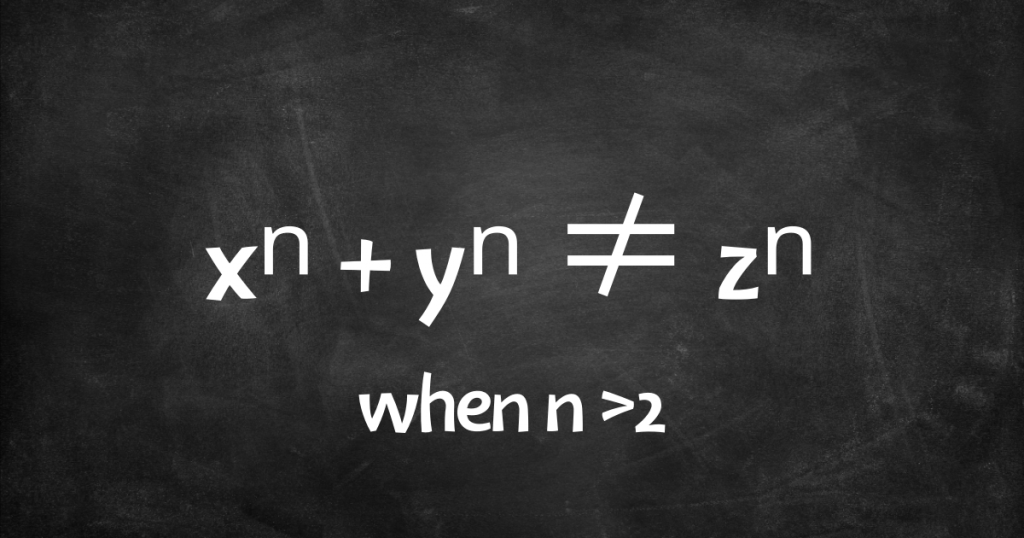
We can find infinitely many solutions that can solve the equations $x+y=z$ and $x^2+y^2=z^2$ in integers, but what about the equation $x^3+y^3=z^3$ or more generally $x^n+y^n=z^n$ , where n is an integer greater than 2 and x,y, z are non-zero integers? Well no one has ever found a single solution of this equation and over three centuries many tried to prove that there is none. Mathematicians like Euler, Legendre, Abel, Gauss, Dirichlet, Cauchy, Kummer, Kronecker and Hilbert put tremendous effort to prove it but failed at some stage.

About a thousand years ago, Arab mathematicians gave a incorrect prove that there were no solutions when n=3. The problem remained untouched until 1637, when the French mathematicians Pierre de Fermat wrote in the margin of a book, “It is impossible to separate a cube into two cubes, a fourth power into two fourth powers, or, generally, any power above the second to into two powers of the same degree. I have discovered a truly marvellous demonstration (of this general theorem) which this margin is too narrow to contain.”

Because Fermat gave no proof, many mathematicians tried to prove the result. The case when n=3 was done by Euler in 1770, although his proof was incomplete. The case when n=4 is elementary and was proved by Fermat himself. The case when n=5 was done in 1825 by Dirichlet and Legendre. The next case of interest was when n=7. This case resisted the efforts of the best mathematicians until it was done be Gabriel Lame in 1839. In 1847, Lame announced that he had completely solve the problem. His approach was to factor the expression, $x^p+y^p$ , where p is an odd prime into $(x+y)(x+ay)(x+a^{p-1}y)$ , where a is the complex number cos(2π/p) +isin(2π/p). Thus his factorization took place in the ring $Z[a]={b_0+b_1a+ldots+b_{p-1}a^{p-1}mid b in Z}$ .

But Lame made the mistake of assuming that in such a ring, factorization into the product of irreducible is unique. In fact, three years earlier, Ernst Edward Kummer had proved that this is not always the case . Within a few week of Lame announcement, Kummer had shown that Fermat’s last theorem is true for all primes of a special type called regular primes. This proves that this theorem is true for for all exponents less than 100, whether prime or not except for 37, 59, 67 and 74. Kummer’s work had developed to the theory of ideals.

Over the centuries many proofs were proposed but failed. Famous Number Theorist Edmund Landau received so many of these for scrutiny and he had a form ready to reply with “On page ____, lines ____ to ____, you will find there is a mistake.”

In March 1988, newspapers and scientific publications worldwide carried news of a proof by Yoichi Miyaoka. However, within a week his proof was shown to be invalid.

In 1993, Andrew Wiles of Princeton University electrified the mathematics community by announcing that he had proved the Fermat’s Last Theorem after 7 years of effort. The Princeton Mathematics department chairperson said. “When we heard it, people started walking on air.” Wiles’ achievement in newspaper and magazine around the world. The New York Times ran a front-page on it and one TV network announced it on the evening news. Wiles even made People magazine’s list of the 25 most intriguing people of 1993. In San Francisco a group of mathematicians rented a 1200-seat movie theatre and sold tickets for $5.00 each for public lectures on this proof. But once again the proof did not hold up under scrutiny.

In September 1994 after a long wait of 358 years, a paper by Wiles and Richard Taylor, former student of Wiles circumvented the gap in the original proof. Since than many experts have checked the proof and found no error. One mathematician said, “The exuberance is back.” However, the proof is quite complicated and fills more than a 100 pages. This proof is one of the biggest mathematical achievements of recent times, and won Wiles many accolades including a special prize at the International Congress of Mathematicians (ICM) in 1998.

References:

J. A. Gallian, Contemporary Abstract Algebra, Narosa.

[The author is an executive member of Gonit Sora and is a fourth year undergraduate student at the Department of Mathematical Sciences, Tezpur University.]

[Editor’s Note: For a good discussion of Kummer’s proof, you can read the article by our Editor of the english section here.]

Sign up for more like this.